数学模拟题

　　Question 1: If a§b = 2a - 3b for all real numbers a and b, what is 2(3§2)?

　　Question 2: A regular polygon has 6 sides. What is the degree measure of the angle, within the polygon, between any two sides?

　　Question 3: A cubic box 'A' has sides of length a and another cubic box 'B' has sides of length 3a. How many boxes A could fit into a single box B?

　　Question 4: If a two-sided coin is flipped 4 times, what is the probability that at least one head will show up?

　　Question 5: What is the value of (2x + 1 - 2x) / (2x - 2x - 1)?

　　Question 6: Side AB of the ABCD square is 10, AE = ED and BF = FC. What is the ratio between the area of parallelogram BFDE and the area of triangle AEB?

　　Question 7: If 15% of x is 45, what is 20% of x?

　　Question 8: Given the | x - 3 | = 5 equation, what is the average of its solutions?

　　Question 9: In the x, y plane, what is the area of the quadrilateral delimited by the x axis, the y axis, the x = 5 line and the y = -5 line?

　　Question 10: If the average of x and y is 3, what is the average of x, y and 6?

参答案

　　参考答案:

　　SAT考试Grid-in练习题3参考答案

　　Question 1: If a§b = 2a - 3b for all real numbers a and b, what is 2(3§2)?

　　Answer: 1

　　Explanation: 2(3§2) = 2(2·3 - 3·2) = 20 = 1

　　Question 2: A regular polygon has 6 sides. What is the degree measure of the angle, within the polygon, between any two sides?

　　Answer: 120

　　Explanation: The sum of all of the angles of a polygon is (n - 2)·180o, where n is the number of sides of the polygon. For a polygon with 6 sides, this is (6 - 2)·180o = 720o

　　The polygon is regular, so all angles are congruent. The degree measure of the angle, within the polygon, between any two sides is 720o/6 = 120o.

　　Question 3: A cubic box 'A' has sides of length a and another cubic box 'B' has sides of length 3·a. How many boxes 'A' could fit into a single box 'B'?

　　Answer: 27

　　Explanation: The volume of box 'A' is a3 while the volume of box 'B' is (3·a)3.

　　The number of boxes A that could fit into a box B is the ratio between the volumes of the 2 boxes. This is (3·a)3 / a3 = 27

　　Question 4: If a two-sided coin is flipped 4 times, what is the probability that at least one head will show up?

　　Answer: 15/16

　　Explanation:

　　p1 = the probability that at least one head will show up when the coin is flipped 4 times

　　p2 = the probability that no heads will show up when the coin is flipped 4 times

　　p1 + p2 = 1

　　The probability that no heads will show up when the coin is flipped 4 times =

　　the probability that the head will not show up when the coin is flipped the 1st time OR

　　the head will not show up when the coin is flipped the 2nd time OR

　　the head will not show up when the coin is flipped the 3rd time OR

　　the head will not show up when the coin is flipped the 4th time.

　　The probability that no heads will show up when the coin is flipped 4 times, p2 = (1/2) ·(1/2) ·(1/2) ·(1/2) = 1/16

　　p1 = 1 - p2 = 1 - (1/16) = 15/16

　　Question 5: What is the value of (2x + 1 - 2x) / (2x - 2x - 1)?

　　Answer: 2

　　Explanation: (2x + 1 - 2x) / (2x - 2x - 1) = 2·(2x - 2x - 1) / (2x - 2x - 1) = 2

　　Question 6: Side AB of the ABCD square is 10, AE = ED and BF = FC. What is the ratio between the area of parallelogram BFDE and the area of triangle AEB?

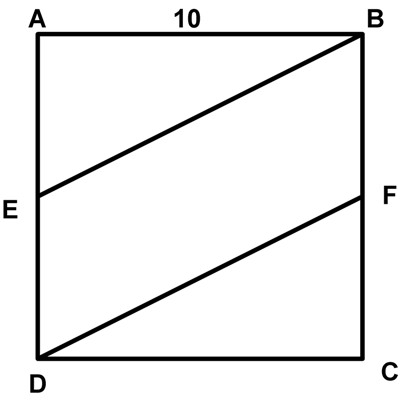
　　Answer: 2

　　Explanation: The area of triangle AEB A1 = (1/2) · AB · AE = (1/2) · 5 · 10 = 25.

　　The area of triangle CDF A2 = (1/2) · FC · DC = (1/2) · 5 · 10 = 25.

　　The area of square ABCD A3 = AB · AD = 10 · 10 = 100.

　　The area of parallelogram BFDE = A3 - A1 - A2 = 100 - 25 - 25 = 50

　　A3 / A1 = 50 / 25 = 2

　　Question 7: If 15% of x is 45, what is 20% of x?

　　Answer: 60

　　Explanation: 15% of x is (15/100)·x

　　(15/100)·x = 45

　　x = 45·(100/15) = 300

　　The problem asks you to calculate 20% of x: 20%(300) = 60

　　Question 8: Given the | x - 3 | = 5 equation, what is the average of its solutions?

　　Answer: 3

　　Explanation: Since |x - 3| = 5, the value of x - 3 is either 5 or -5.

　　x - 3 = 5

　　x = 8

　　x - 3 = -5

　　x = -2

　　The two values of x that satisfy the equation are 8 and -2 and their average is 3.

　　Question 9: In the x, y plane, what is the area of the quadrilateral delimited by the x axis, the y axis, the x = 5 line and the y = -5 line?

　　Answer: 25

　　Explanation:

　　The geometric figure delimited by the 4 given lines is a square with the side equal to 5.

　　The area of the square is 52 = 25.

　　Question 10: If the average of x and y is 3, what is the average of x, y and 6?

　　Answer: 3

　　Explanation:

　　(x + y)/2 = 3

　　x + y = 6

　　(x + y + 6)/3 = (6 + 6)/3 = 4