Angle CMB = Angle AMD

Angle AMD + Angle CMA = 180°

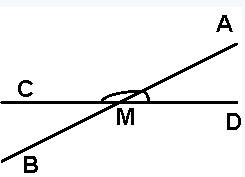
Example of SAT question with angles:In the figure below, angle AMB is 100°, angle BMD is 40° and angle CME is 60°. What is the value of angle CMD?
Figure not drawn to scale

(a) 20°
(b) 30°

(c) 40°

(d) 50°
(e) 80°

Answer: If angle AMB is 100°, then angle BME is 180° - 100° = 80°
BMC + CMD = 40°
BMC + CMD + DME = 80°
If we subtract the 2 equations, DME = 40°
CMD + DME = 60°BMC + CMD + DME = 80°
If we subtract the 2 equations, BMC = 20°
CMD = BME - BMC - DME = 80° - 40° - 20° = 20°

Parallel Lines Review

If the 2 horizontal lines are parallel,
- angles 1 = 3 = 5 = 7 and
- angles 2 = 4 = 6 = 8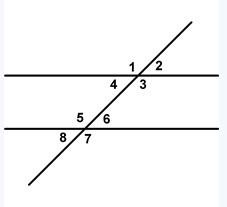

Polygons Review

The sum of the measures of the interior angles of a triangle is 180°.
a + b + c = 180°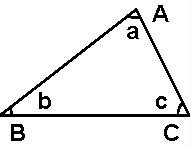

The sum of the measures of the interior angles of a polygon is:
(n - 2)·180°
n = number of sides of the polygon.

The sum of the measures of the interior angles of the polygon above is (5 - 2)·180° = 540°

AC2 = AB2 + BC2.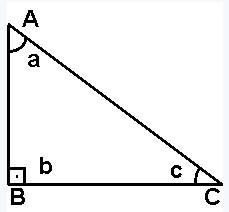

Pythagorean Theorem applied to the triangle above: AC2 = AB2 + BC2.

Equilateral triangle.
a = b = c = 60°
AB = BC = CA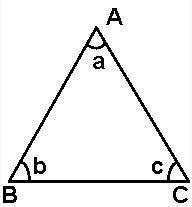

Isosceles triangle.
b = c
AB = AC

a> c > b.
BC > AB > AC

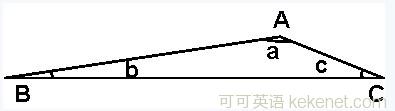
In any triangle, the longest side is opposite the largest angle, and the shortest side is opposite the smallest angle.

Area, Perimeter and Volume

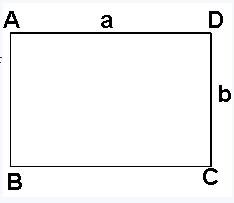
Rectangle Perimeter
2·a + 2·b

Rectangle Area
a·b

Triangle Perimeter
AB + BC + CA

Triangle Area
(h/2)·BC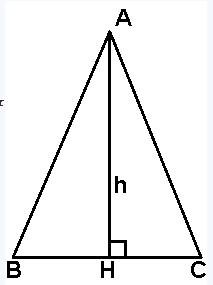

Circle Perimeter
2·¶·r

Circle Area
¶·r2

Cube Volume
a3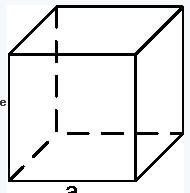

Cylinder Volume
h·¶·r2

　　以上就是SAT数学：几何部分常用公式的详细内容，考生可针对文中介绍的方法进行有针对性的备考。