数学试题

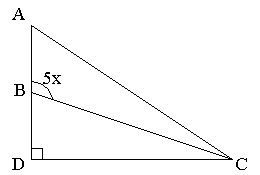
☆1. Which of the following could be a value of x, in the diagram above?

A. 10

B. 20

C. 40

D. 50

E. any of the above

☆2. Helpers are needed to prepare for the fete. Each helper can make either 2 large cakes or 35 small cakes per hour. The kitchen is available for 3 hours and 20 large cakes and 700 small cakes are needed. How many helpers are required?

A. 10

B. 15

C. 20

D. 25

E. 30

☆3. Jo's collection contains US, Indian and British stamps. If the ratio of US to Indian stamps is 5 to 2 and the ratio of Indian to British stamps is 5 to 1, what is the ratio of US to British stamps?

A. 5 : 1

B. 10 : 5

C. 15 : 2

D. 20 : 2

E. 25 : 2

☆4. A 3 by 4 rectangle is inscribed in circle. What is the circumference of the circle?

A. 2.5π

B. 3π

C. 5π

D. 4π

E. 10π

☆5. Two sets of 4 consecutive positive integers have exactly one integer in common. The sum of the integers in the set with greater numbers is how much greater than the sum of the integers in the other set?

A. 4

B. 7

C. 8

D. 12

E. it cannot be determined from the information given.

☆6. If f(x) = (x + 2) / (x-2) for all integers except x=2, which of the following has the greatest value?

A. f(-1)

B. f(0)

C. f(1)

D. f(3)

E. f(4)

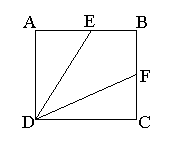
☆7. ABCD is a square of side 3, and E and F are the mid points of sides AB and BC respectively. What is the area of the quadrilateral EBFD ?

A. 2.25

B. 3

C. 4

D. 4.5

E. 6

☆8. If n ≠ 0, which of the following must be greater than n?

I 2n

II n²

III 2 - n

A. I only

B. II only

C. I and II only

D. II and III only

E. None

☆9. After being dropped a certain ball always bounces back to 2/5 of the height of its previous bounce. After the first bounce it reaches a height of 125 inches. How high (in inches) will it reach after its fourth bounce?

A. 20

B. 15

C. 8

D. 5

E. 3.2

☆10. n and p are integers greater than 1

5n is the square of a number

75np is the cube of a number.

The smallest value for n + p is

A. 14

B. 18

C. 20

D. 30

E. 50参考答案

SAT考试数学练习题参考答案

☆1.Correct Answer: B

Explanation:

The marked angle, ABC must be more than 90 degrees because it is the external angle of triangle BDC, and must be equal to the sum of angles BDC (90) and DCB.

Also ABC is not a straight line and must be less than 180.

Therefore 90 < 5x < 180

The only value of x which satisfies this relation is 20.

☆2.Correct Answer: A

Explanation:

20 large cakes will require the equivalent of 10 helpers working for one hour. 700 small cakes will require the equivalent of 20 helpers working for one hour. This means if only one hour were available we would need 30 helpers. But since three hours are available we can use 10 helpers.

☆3.Correct Answer: E

Explanation:

Indian stamps are common to both ratios. Multiply both ratios by factors such that the Indian stamps are represented by the same number.

US : Indian = 5 : 2, and Indian : British = 5 : 1. Multiply the first by 5, and the second by 2.

Now US : Indian = 25 : 10, and Indian : British = 10 : 2

Hence the two ratios can be combined and US : British = 25 : 2

☆4.Correct Answer: C

Explanation:

Draw the diagram. The diagonal of the rectangle is the diameter of the circle. The diagonal is the hypotenuse of a 3,4,5 triangle, and is therefore, 5.

Circumference = π.diameter = 5π

☆5.Correct Answer: D

Explanation:

If two sets of four consecutive integers have one integer in common, the total in the combined set is 7., and we can write the sets as

n + (n + 1) + (n + 2) + (n + 3 ) and

(n + 3) + (n + 4) + (n + 5) + (n + 6)

Note that each term in the second set is 3 more than the equivalent term in the first set. Since there are four terms the total of the differences will be 4 x 3 = 12

☆6.Correct Answer: D

Explanation:

You can solve this by back solving – substitute the answer choices in the expression and see which gives the greatest value.sat

A (-1 + 2) / (-1-2) = -2 / 2 = -1;

B (0 + 2) / (0-2) = 2/ -2 = -1;

C (1 + 2) / (1-2) = 3/-1 = -3;

D (3 + 2) / (3-2) = 5/1 = 5;

E (4+ 2) / (4-2) = 6/2 = 3

If you had just chosen the largest value for x you would have been wrong. So although it looks a long method, it is actually quick and accurate since the numbers are really simple and you can do the math in your head.

☆7.Correct Answer: D

Explanation:

(Total area of square - sum of the areas of triangles ADE and DCF) will give the area of the quadrilateral

9 - (2 x ½ x 3 x 1.5) = 4.5

☆8.Correct Answer: E

Explanation:

Remember that n could be positive negative or a fraction. Try out a few cases:

In case I, if n is -1, then 2n is less than n.

In case II, if n is a fraction such as ½ then n2 will be less than n.

In case III, if n is 2, then 2-n = 0, which is less than n.

Therefore, none of the choices must be greater than n

☆9.Correct Answer: C

Explanation:

If after each bounce it reaches 2/5 of the previous height, then after the second bounce it will reach 2/5 x 125. After the third it will reach 2/5 x 2/5 x 125. After the fourth it will reach 2/5 x 2/5 x 2/5 x 125. This cancels down to 2 x 2 x 2 = 8

☆10.Correct Answer: A

Explanation:

The smallest value for n such that 5n is a square is 5.

75np can now be written as 75 x 5 x p.

This gives prime factors.... 3 x 5 x 5 x 5 x p

To make the expression a perfect cube, p will have to have factors 3 x 3 , and hence p =9

n + p = 5 + 9 = 14